
Meanwhile, exhaust velocity has increased, but not enough to overcome the growth in the second term. The nozzle is now sucking on the atmosphere. If we further extend the nozzle, the exit pressure drops below ambient, and the second term goes negative. Obviously, we need to consider equations other than just the thrust equation to determine this, but that's how it works out. In fact, the increase in velocity more than offsets the the loss in the second term. Now let's lengthen the nozzle to the point that the exit pressure drops to ambient. The exhaust gas at the exit is pushing on the atmosphere, and that helps generate thrust. So, the second term in the thrust equation is positive. Now, let's do the thought experiment of starting up a rocket engine that's underexpanded at some non-zero ambient pressure.

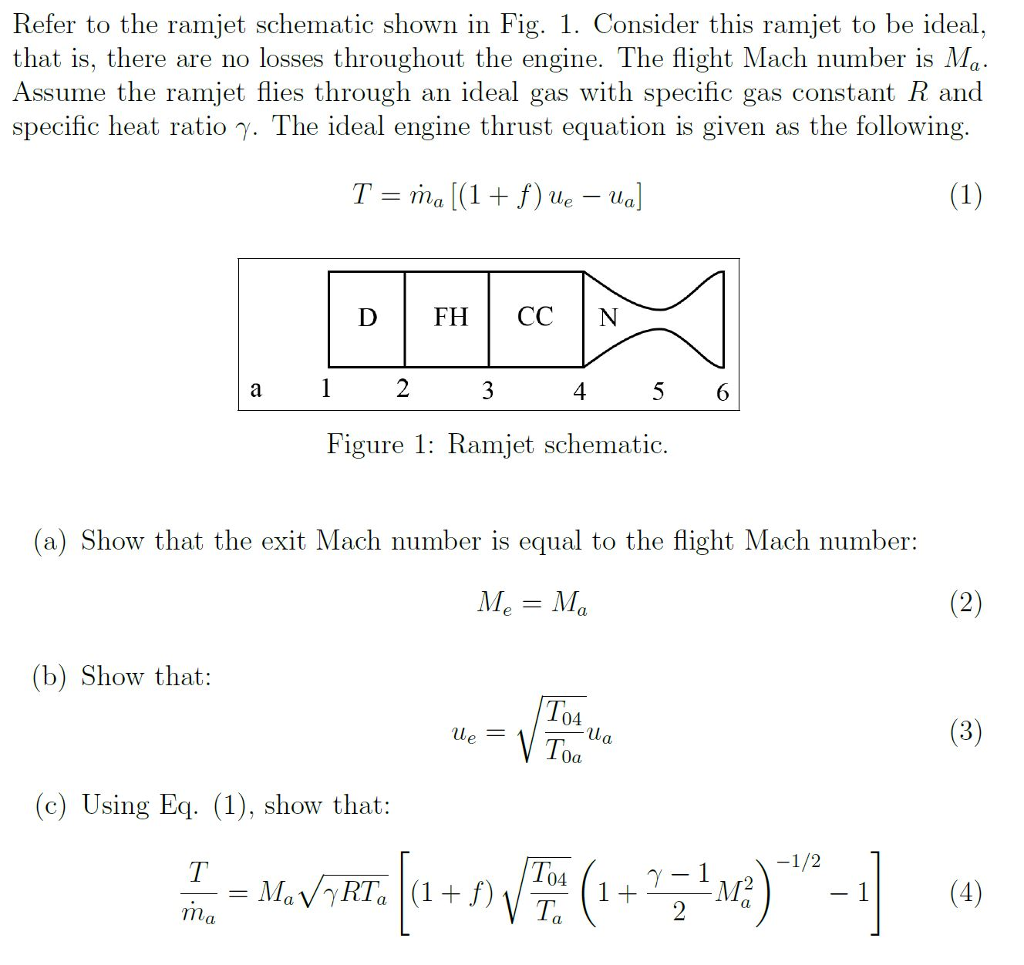
Where q is the mass flow rate (usually represented by m-dot, of course), v e is the exit velocity, A e is the exit area, p e is the exit pressure, and p a is the ambient pressure. In the simple one-dimensional-flow model of a rocket engine, thrust is equal to Now, when it comes to rocket engines outside the atmosphere, is it the lack of ambient pressure which allows us to utilize large throat to nozzle area ratios (ignoring practical constraints), and thus reducing the pressure ratio requirements to obtain high efficiencies (except where it significantly affects combustion temps)?

However, it's the divergent section of a nozzle where the potential energy of the gas is converted to kinetic energy (reducing the gas temperature in the process), correct? Since a higher PR allows for a higher throat to nozzle exit area ratio (exit area being determined by ambient pressure), more of the potential energy can be extracted in the expansion phase, right? Is it safe to say that any heat not absorbed by the expansion process, and thus above ambient temperature is a waste? If we keep the combustion temperature between the two engines the same, is the increased efficiency of the engine with the higher PR derived primarily from the nozzle, specifically because it allows a higher throat to nozzle exit area ratio? I understand (hopefully correctly) that mass flow rate through a nozzle throat can be determined by the upstream pressure, so for a given throat area, an engine with a higher pressure ratio will have a higher flow rate. It's generally assumed the engine with the higher PR will have better efficiency (so more thrust for a given mass flow rate, or better SFC for a given thrust level). Lets say we have two (ram or turbo)jet engines - one operates at a pressure ratio of 10 while the other operates at a PR of 20. I'm going to state my understanding of the subject, and am hoping that someone will tell me where I'm wrong, and perhaps even show me how thermodynamic concepts like entropy, enthalpy, etc. My question is more focused on jet engines, but I assume the concept holds true for rocket engines operating in an atmosphere. Hopefully I'll be welcomed, and you won't all be too hard on me
Since I've come across this forum numerous times while searching various topics and saw how many smart people post here, I figured this might be a good place to get some clarification on my questions. I've also read some explanations on other forums but some of them leave me with the feeling that they're wrong. Similar coatings and similar interdiffusion calculations might have broad applications.I know this topic has been addressed all over the web, but as someone who doesn't really have a grasp on the concepts and terminology of thermodynamics nor calculus, seeing explanations such as "Because formula X(YZ) says so" doesn't really help me, unfortunately. This would cost approximately $60,000 per reactor unit and should more than double reactor lifetime.
This coating is expected to catalyze the reaction D m is recommended. Thin palladium coatings on the outside of the zirconium are analyzed as a method for deuterium removal. This deuterium weakens the tubes through hydrogen embrittlement. This paper reports that, in pressurized heavy water nuclear reactors of the type standardly used in Canada (Canada deuterium uranium-pressurized heavy water reactors), the zirconium alloy pressure tubes of the core absorb deuterium produced by corrosion reactions.


 0 kommentar(er)
0 kommentar(er)
